Equal Chords and their Distances from the Centre
Equal Chords and their Distances from the Centre: Overview
This topic contains concepts like Properties Related to Chord of a Circle, Equal Chords and their Distances from the Centre.
Important Questions on Equal Chords and their Distances from the Centre
In a circle with radius , two equal chords are at a distance of from the centre. If the lengths of the chords is , then find the value of .
Two equal chords and of a circle intersect at a point within a circle then

Two equal chords and of a circle intersect at a point within a circle then
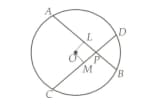
Two equal chords and of a circle intersect at a point within a circle then

Three chords and of a circle are respectively and away from the center. Which of the following is correct?
Which of the following statements is true for the longest chord of a circle?
Prove that, of any two chords of a circle, the greater chord is nearer to the centre.
Circles with centres and intersect at points and as shown in the figure. is a line segment and is tangent to the circle, with centre at point If the circles are congruent, show that
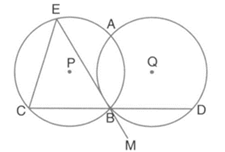
Two congruent circles of centres and intersects each other at point and , then prove that .
If two chords and are away from the centre of a circle, then .
The chords of a circle of length and . Which apart from the centre are and respectively.
Chords of a circle and are and , which makes angle at centre are of and respectively.
In the figure below, and are two equal chords of a circle and is the center of circle. If and , then prove that .
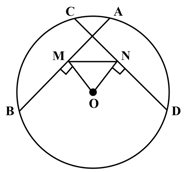
Prove that out of all chords which passes through any point of circle, that chord will be smallest which is perpendicular on diameter which passes through that point .
If two equal chords of a circle intersect each other, then prove that two parts of a chord are equal to other corresponding both part of chord.
In a circle the chords are at same distance from the centre, then one chord is _____ of(to) the other.
